Lending Insurance Decentralised Options Vaults (LIDOV)
The Use of Options Contracts to Secure Loans in Money MarketsAbstract
DeFi money markets face many challenges in scaling to significant levels, due to their capital inefficiency and their high-risk nature. Current methods of reducing risk in DeFi money markets require considerable overcollateralisation and insurance reserves, which are limited in both their effectiveness and efficiency. This paper outlines how Decentralised Options Vaults (DOVs) can function as insurance for lenders, optimise yields, increase capital efficiency of markets, and reduce overall risk.
1. Introduction
The anonymity of Decentralised Finance (DeFi) forces overcollateralisation of loans, as lenders are unable to assess counterparty risk.
Despite being overcollateralised, DeFi loans present several risks associated with the volatility and illiquidity of the provided collateral they receive. If the value of a loan’s collateral drops too rapidly, lenders will struggle to get back their capital, leading to insolvency and bad debt in the market. Currently, this risk of insolvency is reduced by sacrificing capital efficiency.
There are two common methods to reduce insolvency risk in DeFi. The first is to include a reserve fee (or reserve factor) in the money markets transactions, which acts as insurance by building a reserve of capital to draw from in case borrowers can default on their loans. The second method is to reduce utilisation rates, which is the ratio between the quantity of assets supplied and the quantity of assets borrowed. Both methods leave assets sitting idle in protocols, while only offering limited on-chain guarantees against insolvency.
1.1 Insurance Reserves
Traditional lending protocols such as AAVE or Compound use a reserve pool model to hedge the risk of insolvency. This model usually involves taking a commission on the lender’s interest rate, called a reserve factor. The commission decreases the interest rate received by lenders, redirecting part of the yield to a reserve. The reserve is intended to hold a quantity of the asset supplied by lenders, and grow over time through commissions. It can be called upon by the protocol to repay bad debt.
1.1.1 Utilisation rates
To make certain that lenders can always withdraw deposited funds, money market protocols set optimal utilisation rates. These rates determine how much of deposited funds should ideally be issued as debt to borrowers.
As the utilisation rate approaches 100%, lenders are increasingly unable to withdraw their deposits. To protect lenders, protocols aim to keep utilisation rates low, reducing the likelihood of a bank run. Lower utilisation means that funds are sitting in the market idle, remaining available for lenders wishing to withdraw.
Idle funds in the market represent capital inefficiency, as this capital deposited by lenders is not able to earn interest if it cannot be borrowed. Traditional lending protocols thus decide between capital efficiency and risk. Optimal utilisation rates define a protocol’s strategy to balance this tradeoff.

1.1.2 Low capital efficiency
The insurance reserve model requires a significant amount of capital to be locked away, resulting in capital inefficiency and opportunity cost. These funds represent yield, and thus value, lost by the protocol, which could’ve gone to lenders. Instead these assets are inactive in order to provide security for lenders in case of future insolvency. The direct value in the form of yield is now made indirect in the form of capital protection. With a reserve factor, the protocol transforms part of its tangible value to lenders into something intangible.
Intangible value is not less valuable, but remains problematic as it is harder to advertise and measure.
1.1.3 Latency
Markets require high amounts of churn before the commissions from the interest rate build up into a significantly sized reserve. This leaves lending markets exposed to higher risk at launch when they have no reserves yet. The risk decreases over time as the reserve grows. While new markets and collaterals are the riskiest and require the most protection, the reserve model proves to be least useful during this period where it is the most needed. It only builds up significant capital once markets have matured and the overall risk is already reduced.
Risk protection for lending markets should ideally be dynamic, to adjust to the latest risks that arise for each collateral. A dynamic model could enforce stronger protection in the earliest days of a market’s lifecycle, then optimise for capital efficiency as it matures. The reserve model however is linear in how it accrues capital, and thus cannot accurately and promptly address the current levels of risk for each asset.
1.1.4 Low coverage
If a market continues to grow, the reserve model cannot protect large amounts of debt.
The churn from borrowers sending a fraction of their interest into a reserve can rarely outpace the speed at which debt enters a market. This makes it difficult for the reserve to insure a significant amount of the outstanding debt.
2. Lending Insurance Decentralised Options Vaults
Instead of losing yield in the protocol by sending it to a reserve, option vaults serve as an opportunity to redirect that yield and pass it on to different stakeholders. Through their active participation, these stakeholders are meant to be more effective than the passive role of the traditional reserve.
Options contracts known as cash secured puts can be used to incentivise such stakeholders, and how their inclusion in money markets makes for both a more effective and more efficient method of insurance.
2.1 Cash Secured Puts
Cash Secured Puts as they exist in traditional finance hedge an asset’s downside risk.
Like other options, they are contracts between buyers and sellers. The buyer is purchasing the right (or option) to sell an asset at a fixed price but at a future date. Inversely, the option seller is selling a guarantee that they will purchase said asset from the buyer at that fixed price on the agreed upon date. Depending on the price at the agreed upon date, either the buyer or seller stands to make a profit on the sale.

It’s impossible to know for sure if the price of the asset will be below or above the strike price when the contract expires. The seller is compensated for this uncertainty by receiving a regular payment from the buyer known as an option premium.
By purchasing the Cash Secured Put, the buyer has a guarantee that they can sell the asset that they hold at the strike price, which limits their potential losses. In other words, the buyer is purchasing insurance on the price of their asset declining.
2.2 Decentralised Options Vaults
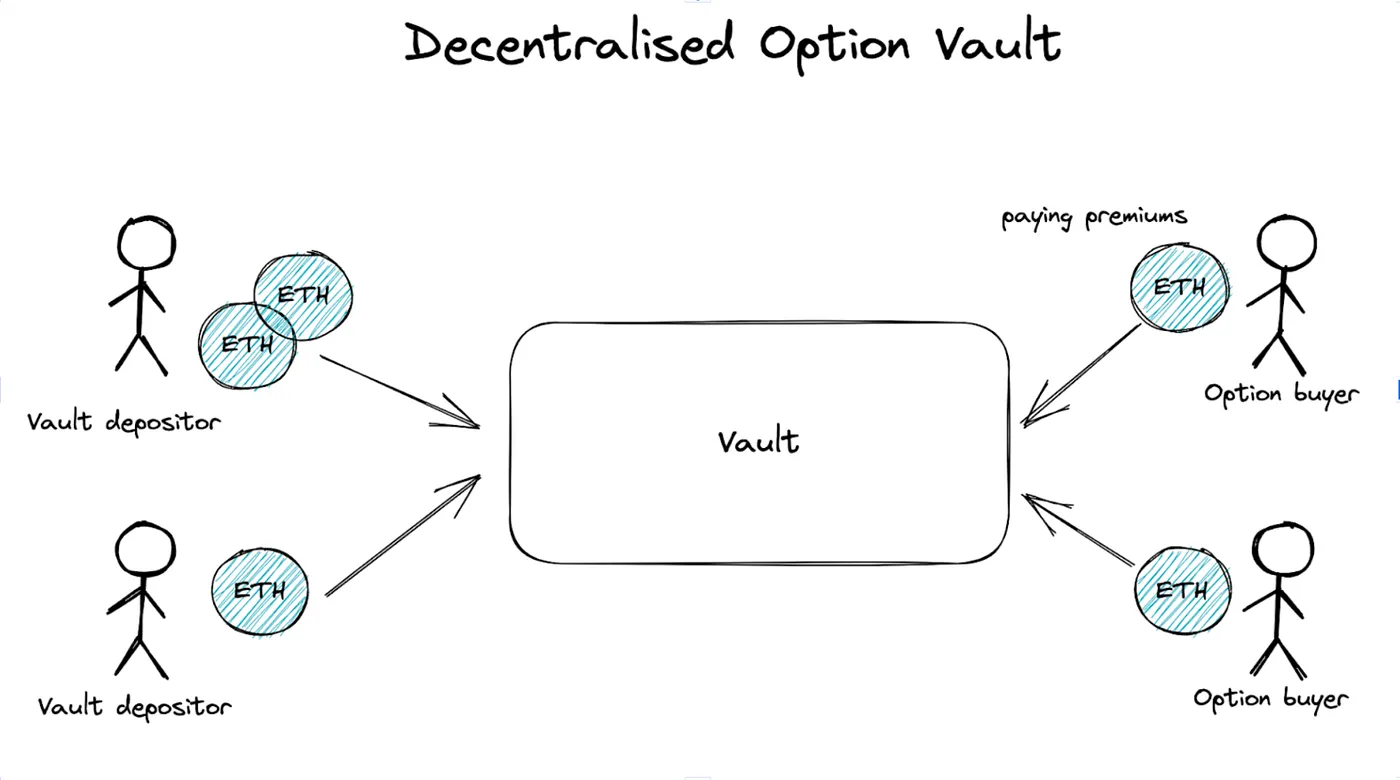
If options contracts share the same terms (duration, strike and underlying asset) buyers or sellers can pool together into a vault, called Decentralised Options Vaults (DOVs), where depositors lock their liquidity for a set period of time.

2.3 Modifying DOVs for lending protocols
Lending Insurance DOVs are a version of DOVs where the options buyers are the lenders of a money market. The underlying asset being insured is the collateral provided by borrowers to lenders, and the premiums paid to sellers come from a commission on the interest rates received by lenders.
2.3.1 Lenders purchasing options
Lenders forfeit part of their interest rate in exchange for the guarantee that the collateral they hold from borrowers can be sold at a fixed price. They exchange a higher, and riskier interest rate, for a lower, but safer rate.
By insuring the downside risk of the collateral in money markets, lenders are safeguarded from bad debt. They also receive a guarantee that the loans are fully solvent thanks to options sellers offering their capital to lenders in the event of collateral prices dropping.
If collateral prices drop below a strike price, protocols can withdraw tokens deposited in the option vaults by the option sellers. This enables loans to remain solvent even as collateral provided by borrowers loses value. If the collateral becomes insolvent, or not enough value can be extracted to pay back lenders, capital can instead be withdrawn from DOVs to pay back lender deposits.

This mechanism emulates a decentralised and on-chain version of Credit Default Swaps (CDSs), in which lenders agree to receive a reduced interest rate on loans, in exchange for fixed or secured yield. CDSs are particularly popular in traditional finance’s long tail markets like mortgage markets, and they have the potential to be even more relevant in DeFi lending markets for long-tail assets such as NFTs and derivatives.
2.3.2 Insurers selling options
Vault depositors in the LIDOVs act as option sellers. While they lock their funds in the vault, they are compensated with premiums paid by lenders, who use the vault for insurance. There are two main reasons why someone would opt to become an option seller, or an insurer as they will henceforth be referred to.
The first reason is they wish to acquire the collateral at a discount from its current market price. This means they speculate that there is a realistic probability for the collateral price to match the strike price set in the option vault, in which case their deposit functions as a buy order. Unlike a traditional buy order, they are being paid to keep this bid open for a set amount of time.
The second reason is if they wish to earn yield in the short term, by speculating on the price of the collateral. If they believe it’s unlikely for the collateral price to reach its strike, then the option vault presents a simple yield opportunity. In the worst-case scenario, they are left holding the collateral associated with their vault.
How much of their vault deposits can be reclaimed by insurers in the case of the option being exercised would depend on how much capital is needed to repay bad debt in the market. Vault depositors can withdraw any remaining liquidity in the vault once lenders are made whole on their insurance.
2.3.3 Automating premium pay-outs
LIDOVs offer the advantage of automating parts of the option vault flow. Whereas a DOV protocol usually finds buyers and sellers of its contracts on options markets, a LIDOV can be integrated natively into a lending protocol. Lenders automatically become options buyers, simply by accepting a reduced interest rate.
This commission on their interest rate is accrued into a temporary reserve and sent by the protocol to the vault at the beginning of its cycle. If a vault requires insurers to lock capital for a duration of 1 week, the protocol must have accrued enough in its reserves to pay-out the premiums at the beginning of the week. If limited interest has accrued in lending markets in the previous week, then promised pay-outs will be smaller for next cycle’s vault depositors, meaning options buyers must accept a less favourable strike price.
3. Benefits of LIDOVs over reserve pools
Paying the premiums on options instead of pooling funds has a few advantages, which directly mirror the disadvantages of the reserve pool model.
3.1 Capital efficiency of options
By removing the need to hold assets sitting idle in reserve pools, LIDOVs improve capital efficiency in DeFi protocols, using no more than the amount of funds required to offer insurance, and leaving the rest to lenders.
3.1.1 Forwarding lost yield to new stakeholders
The first way LIDOVs improve upon capital efficiency, is by deploying 100% of the assets accumulated in the insurance reserve. Instead of having yield build up into a reserve, it is redirected to different stakeholders, and thus not lost in the short term.
Whereas an insurance reserve pool holds assets for a hypothetical decline in prices, a LIDOV redirects the yield, and keeps liquidity flowing in the protocol by creating a new category of stakeholders: options sellers.
3.1.2 High utilisation rate due to lower risk
Capital efficiency is not simply found in the full utilisation of reserve assets, but also in the utilisation of deposited funds. Because a LIDOV guarantees that part of a lender’s deposit can be recouped, the risk in the market is significantly reduced, resulting in higher utilisation rates.
This guaranteed insurance for lenders on their deposits, which emulates institutions such as the FDIC, reduces the need for lenders to withdraw funds in market downturns. While utilisation rates are usually kept low to prevent bank runs, the guarantees offered by LIDOVs ensure that lenders can recoup deposited funds, enabling utilisation rates to be set higher. The higher utilisation means more capital from lenders is being deployed and earning yield.
3.2 Low latency
LIDOVs can also be deployed as a market is created. While an insurance reserve requires churn from borrowers entering and exiting the market to build up significant coverage, LIDOVs can be deployed almost immediately.
The protocol can decide to fund the LIDOV’s first cycle before any interest has accrued. Or it can wait before enough interest is accruing to begin funding options premiums. Thus, LIDOVs are a solution for listing novel assets in lending markets whilst protecting users in the market’s early days.
3.3 High coverage, creating a leverage effect.
By utilising options contracts, LIDOVs are able to cover more debt with the same amount of capital, thanks to the leverage provided by options. Whereas a reserve pool with 100 ETH in reserves could cover 100 ETH worth of bad debt in a market, that same pool of capital could cover between 1,000 to 10,000 ETH of bad debt if it were to be deployed in options. Or vice versa, covering 100 ETH for the duration of the LIDOV could only end up costing the protocol 1 to 10 ETH in options premiums.
This means LIDOVs offer more efficient insurance coverage, relative to how much leverage can be obtained on the underlying options which makeup the vault. They can more easily cover the entirety of the outstanding debt within a market, something that is unfeasible with the reserve pool model.
3.4 Adjustable coverage
Not only is coverage extensive and efficient, it is also adjustable. If a LIDOV has a duration and frequency of 1 week, markets can adjust insurance on a weekly basis. When risk for a collateral asset increases, its corresponding LIDOV can be adjusted to select higher strike prices or higher insurance coverage.
Inversely, if the underlying risk of an asset were to decrease, for instance through reduced volatility, premiums and insurance could also be adjusted.
4. Implementation of LIDOVs
Each protocol has its own optimal use for LIDOVs, but below is a general illustration of how insurance vaults could be utilised in an NFT lending protocol, where collateral is usually novel, illiquid and risky.
A LIDOV can exist to protect a group of markets, like cross-margin protocols, or insure individual isolated risk markets (IRMs). In this example, it’s assumed the NFT lending protocol uses IRMs, where each collection has its own dedicated market, liquidity, and LIDOV.
4.1 Strike prices (FFTs)
An important challenge when implementing LIDOVs in NFT lending protocols is tracking the value of the collateral. Normally, a LIDOV sets a strike price on the same assets held by lenders as collateral. If lenders hold asset A as collateral, a strike price is set on asset A to ensure that even if its value decreases, the collateral can remain solvent if sold through the option.
If a LIDOV is used across an entire market, it must treat all collateral the same. To do this, we need to track the value of collateral assets based on their lowest possible price, known as the floor price. In this example, we sacrifice accuracy of pricing for security, underestimating the value of any given collateral to not overestimate the sum.
However, the collateral held by lenders in an NFT lending protocol is non-fungible, and market-wide insurance through a LIDOV assumes fungibility between insured lenders. Fungible Floor Tokens (FFTs) bridge this gap between fungibility and non-fungibility, and establish a base upon which market-wide insurance contracts can be built.
An FFT is a token backed by an arbitrary NFT held within a vault. These vaults will contain multiple NFTs from the same collection, and with each NFT added, a new FFT is minted. In a rational market with sufficient arbitrage, FFTs should track the aggregate value of the NFTs backing them, divided by the number of outstanding FFTs. With a large enough sample of NFTs contained within the vault, the corresponding FFTs create a fungible and tradable reflection of the collection’s floor price.
A LIDOV, which requires a strike price for the asset it insures, would track the market price of FFTs for the corresponding collection.
Vault depositors would supply a native asset or stable token into the vault. If the value of collateral held by lenders drops, said collateral would be swapped into FFTs during a liquidation.
Vault depositors would find themselves holding a certain amount of their deposited assets, and a certain amount of FFTs, the sum of which should be roughly equivalent to the amount deposited into the vault. Depending on the liquidity of these FFTs, vault depositors may incur a loss, but they are compensated for this illiquidity risk through the premiums they receive.
4.2 Insurance coverage
As previously explained, insurance using options can cover between 0 and 100% of the outstanding debt in a market. Therefore, an important consideration for implementing a LIDOV is how much of the outstanding debt should be insured, and what are the metrics which can provide quantitative and data-driven answers.
4.2.1 Intrinsic value
The most obvious consideration when looking at the potential price of an asset should be its intrinsic value. If the asset is backed by yield or collateral, it has an intrinsic value to its holder below which it shouldn’t fall under.
For these kinds of assets, a LIDOV could simply have to cover the difference between the asset’s market price, and its intrinsic value. Any additional coverage would be redundant.
4.2.2 Volatility based probabilities
Volatility of the asset can also be used to estimate the necessary coverage. The average volatility of an asset over the duration of the insurance contract, could be indicative of how much coverage is required for the collateral.
Assuming the normal distribution of volatility around the average price of an asset, we can determine the probability of volatility large enough to make the market undercollateralised.
5. Fixed rate lending implementation
Credit Default Swaps enable another important feature, the ability to distribute fixed yield.
As the name implies, fixed rates provide lenders with a promise that over a certain period, interest rates will not fluctuate. Fixed rates like treasury bills or bonds are fundamental to a financial system yet can be very rare in DeFi.
Lending interest rates in DeFi are usually variable, as they are a function of supply and demand for liquidity within a market.
The swapping of risk between lenders and insurers opens the door for a possible implementation of LIDOVs in which interest rates can be non-variable for the duration of an option contract. This enables lenders to swap their high but variable yields from borrowers, in exchange for fixed yield guaranteed by options sellers.
Conclusion
LIDOVs lay out a new evolution in DeFi money markets which could provid increased stability to yields, higher capital efficiency and wider support for high risk assets. Their exact implementation can vary based on the needs of different protocols. Further research will have to investigate the use of American options over European options, whether fixed yield is more profitable for vault depositors or for lenders, as well as the returns of an insured market relative to the risk free rate.